Tag: cube

Sonobe modules

Some of the Maths & Crafts students volunteered in February to help Trefor Bazett run an origami event as part of a day of math and engineering fun on campus (organized in partnership with Let’s Talk Science). Our event centred on Sonobe modules.

What is a Sonobe module? Many origami geometry models are modular, or based on units (see PHiZZ and Wireframe cube). The Sonobe module is a one-paper unit that is named for Mitsunobu Sonobe. It may have been created by Toshiba Takahama (they were coauthors and members of the same origami group), or it may have been invented by someone else and named for the first person to mention it in a publication (in 1968).
Image of a single Sonobe unit.
Sonobe unit.
Use: Three Sonobe modules lock together to form a tetrahedron corner (much like the PHiZZ module).
Three Sonobe units locked together.

One you have folded some Sonobe units, a good starting model is to assemble them into a cube:

Sonobe cube.

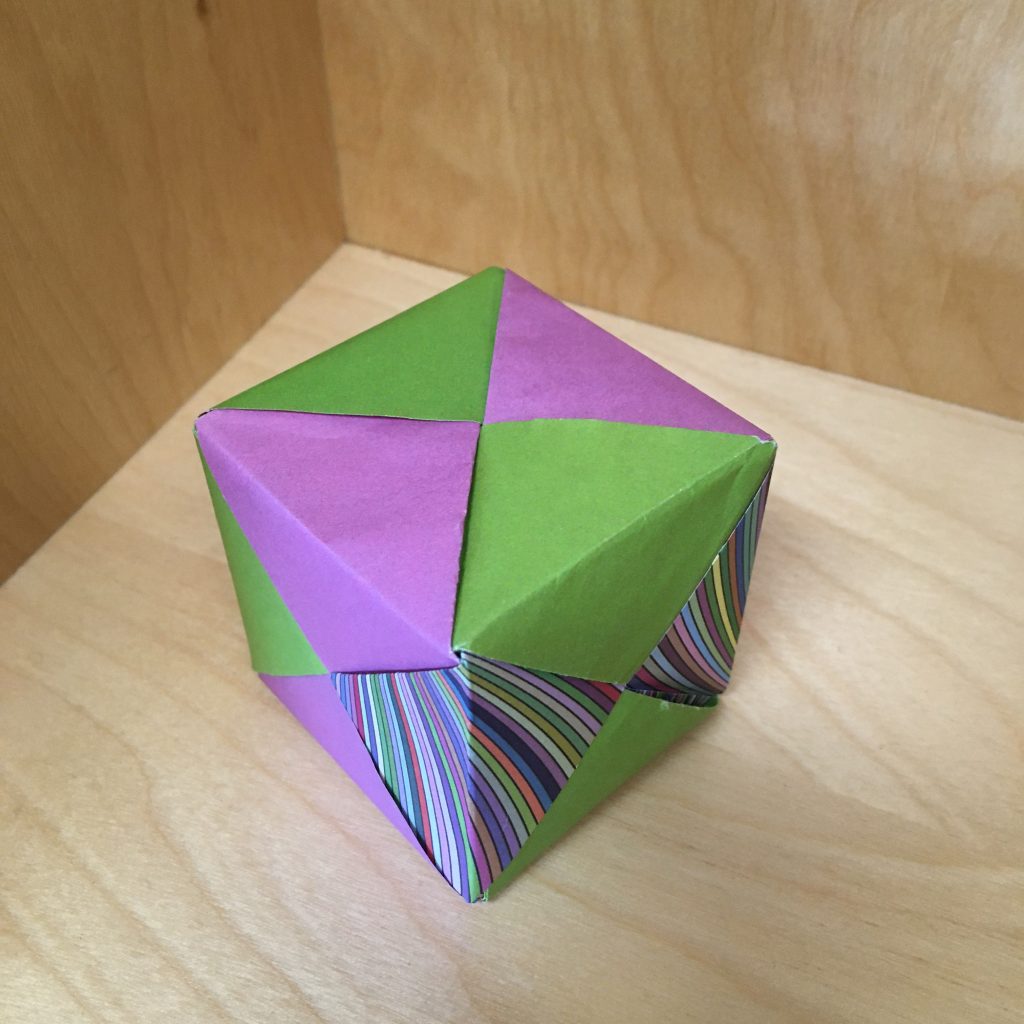
You can build other three-dimensional shapes with them too: a “triakis octahedron” or a “triakis icosahedron”. There are some examples of triakis octahedra in the Elliot building origami display, and there is an image of a triakis icosahedron at the end of this post.

The word “triakis” means that each face of the original shape has been replaced by a tetrahedron, which is the Platonic solid formed by four equilateral triangles. Because each face of an octahedron is an equilateral triangle, as is each face of an icosahedron, those shapes are suitable for the “triakis” treatment.

Questions to ponder (some hints below):

  1. How many Sonobe units do you need to build a cube?
  2. If you wanted to “properly colour” your cube, how many colours would you need?  Here we just mean that two papers of the same colour shouldn’t be attached to each other.
  3. Why is the cube special? The other models are “triakis” things; why isn’t the cube?
  4. How many pieces of paper would a triakis icosahedron need? 
  5. How many colours to properly colour the triakis octahedron? triakis icosahedron? (Three are certainly needed, because the units are assembled in trios.)

Resources from Maths Crafts New Zealand

Hints and answers to my questions:

  1. A cub needs six Sonobe modules. There are at least two ways to count it! First way: each Sonobe module is going to end up with its square inner bit being the face of a cube. How many faces has a cube got? Second way: each triple of Sonobe modules is going to form the corner of a cube; how many corners is each module involved in, and how many corners has a cube got?
  2. You’ll need at least three colours for any of these models, because Sonobe units lock together in trios. For the cube, three colours suffice.
  3. The “cube” is actually a “triakis tetrahedron”, so it’s not as special as it seems! This might take some drawing to work out; you might find it helpful to imagine what is left over if you slice each vertex off of a cube.
  4. An icosahedron has 20 faces, each of which is replaced by a trio of Sonobe units, and each unit participates in two faces, so you need 30 pieces of paper.
  5. For the triakis octahedron and the triakis icosahedron, three colours suffice (the triakis icosahedron pictured below is properly three-coloured). It is harder to properly three-colour the triakis icosahedron, though!
Sonobe triakis icosahedron.

Menger sponge

The Menger Sponge is a three-dimensional fractal curve; if you have seen the Cantor Set (in one dimension) or the Sierpinski Carpet (in two dimensions), the Menger Sponge will look familiar to you. If you haven’t see those other things then this is a good opportunity to spend some quality time on Wikipedia.

In his book Project Origami, Thomas Hull gives instructions for folding models of portions of the Menger Sponge out of business cards. He also includes this dire warning:

Instructors in any class using this activity should be forewarned that it is normal for students to become addicted to making business card cube structures.

For our final mathematical paper folding project of December, we assembled a Level 1 Menger Sponge model:

Photograph of our Menger Sponge business card model.
Our level 1 Menger Sponge, built out of 120 business cards.

This model used up my entire supply of old University of Minnesota business cards; to make the Level 1 sponge we will need another 2,280 business cards. If you have any defective business cards (former institution, old job title) you would like to donate to our cause, please let me know!

The octahedron is dual to the cube

Earlier I shared a video of me folding a cube made from Fuse’s wireframe modules. While I was working at the University of Minnesota’s Math Centre for Educational Programs, I learned a different way of folding a wireframe cube. I don’t know the original designer, because the video I used to reference is gone. Here is a video of me slowly folding the module (in case you want to learn yourself) and then a time-lapse of me assembling it into a cube:

Two hands holding a partially-assembled paper cube.
Click on the image to watch the video.

https://echo360.ca/media/f2f16367-8010-4097-b635-2ab4debbd49d/public

The cube is one of the five platonic solids (named for the ancient Greek philosopher Plato). A platonic solid is a solid whose faces are all the same, whose faces are all regular polygons, and whose vertices all have the same number of faces meeting together. Faces of the cube are regular 4-gons (squares), and at each vertex three faces meet. Now, if you put a vertex in the middle of each face and then connect two vertices together if and only if their corresponding faces share a mutual edge you get another solid. We call that the dual of the first one. It turns out that the dual to a platonic solid is always also a platonic solid. Grab a cube and see if you can work out what its dual is!

Go ahead; I’ll wait.

You could always fold one yourself; you just need 12 pieces of paper!

The shape you have (perhaps) just drawn or visualized is called an octahedron because it has eight faces. Each face is an equilateral triangle. In this next video I’ll show you how to fold a model of an octahedron, which I also learned at the Math Centre for Educational Programs. It’s made out of six “water bomb” modules. While at UMN, I regularly helped teach local teachers how to fold these and help their grades 3 and 4 students assemble the model, as part of the Girls Excel in Math (GEM) program there. That means that this model is not too hard to put together; it is certainly easier than the wireframe cubes.

Watch to the end to see a demonstration of their duality!

Two hands holding a partially-assembled octahedron.
Click on the image to watch the video.

https://echo360.ca/media/e7b2d811-dbd2-4bf6-be4d-9f3082d9a705/public

Folding a cube with wireframe modules

Tomoko Fuse’s wireframe unit (from The Complete Book of Origami Polyhedra) is great for making cubes. This video might help you follow his instructions, and work out how to lock the units together. Watch to the end to see my final product!

Two hands holding a partially-assembled origami cube.
Click on the image to start the video.

https://echo360.ca/media/617d6ee8-d35e-4676-a783-3a1bad7c22f9/public