Category: Uncategorized

Abstract Algebra Everywhere


There is a box containing “N” balls. Each ball has a number: bi; i from 1 to N.
Each ball is colored by one color, and there are “M” different colors. The number “bi” is equal to the number of balls whose color is as same as the color of the ith ball. For instance, if there are 4 red balls each red ball has the number 4.
If the summation of 1/bi from i=1 to N is 10, find M?

Although it is an easy puzzle, the idea of this puzzle is exactly that of counting orbits under a group action, which is presented by George Frobinous (it is usually referred to as Burnside’s Lemma).

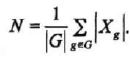
Human VS AI

There is a question that how much artificial intelligence can become close to the human brain. So, the question of what the differences between humans and machines are arises. Sometimes, I think to myself the difference is that we can understand the concept of infinity but machines cannot. Our thoughts can walk at the area of infinity. That is, roughly speaking, we can say the distance between elements of a Cauchy sequence is zero at infinity but not zero everywhere. However, in computers, because of the lack of precision, the distance becomes zero when the distance becomes smaller than the machine epsilon, and even if we would have a computer with infinite precesion, then the distances would never become zero. In another way, there is a difference between finiteness and boundedness. For example, tan(x) is finite between -pi/2<x<pi/2 but not bounded. However, machines cannot realize this difference.

What are the superior tools for having a better cognition of our universe?

Do you ever wonder why Russians are good at chess and math? Maybe, it is related to their language, specifically the structure of their language.

Do you know that Carl Friedrich Gauss-great German Mathematician- was skeptical about studying math or linguistic at the age of 17? Maybe, there is a relation between linguistic and mathematics.

Do you ever think about the triangle of math, linguistic, and logic?